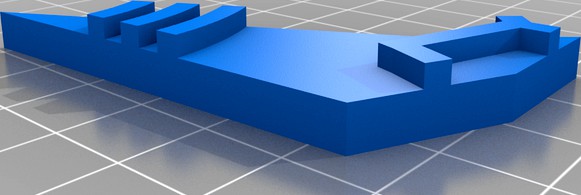
Irregular Tesselating Pentagon Type 6 by claudeShannon

Last crawled date: 3 years, 4 months ago

Irregular Tesselating Pentagon Type 6
John Matz
11/13/2020
George Mason University
Math 401: Mathematics Through 3D Printing
This irregular pentagon is one of the fifteen pentagons that can seamlessly tile the infinite plane. Though this particular tile is concave or nonconvex, it is a type 6 pentagon, which is one of the pentagons that will tessellate as either a convex or concave type. According to Wikipedia, “Types 1, 2, 4, 5, 6, 7, 8, 9, and 13 allow parametric possibilities with nonconvex prototiles.”[1]
The Type 6 pentagon is one of three pentagon types (6, 7 & 8) discovered by Richard Kershner in 1968.[1] He believed that these were the last possible pentagonal tilings, but seven more types were subsequently discovered.[1] Only recently, in July 2017, did Michaël Rao successfully execute a computer-assisted proof that demonstrated that there were no remaining undiscovered convex pentagonal tile types.[1]
The Type 6 pentagon is differentiated from other pentagonal tiles by its conformity to five apparently simple equations[1]:
a = d = e (1)
b = c (3)
B + D = 180° (4)
2B = E (5)
When the internal angle A is greater than 180° the pentagon becomes concave, as mine is. I gained a better understanding of the underlying geometry of this pentagon by dividing it into three triangles and attempting to solve for all of the sides and angles. According to Quanta Magazine, all n-gons are divisible into n-2 triangles, thus all pentagons are divisible into 3 triangles.[2] Defining angle B as 19°, D is then 161° by equation (4), and E is 38° by equation (5). Then, by defining one of the component triangles at that adjacent to point E, it is clear that triangle must be isosceles and it is possible to find the other two angles of this triangle. Starting from this basis, it is possible to solve for all the other angles and side lengths by hand through use of the Law of Sines, the Law of Cosines, and basic trigonometry.
[1] https://en.wikipedia.org/wiki/Pentagonal_tiling
[2] https://www.quantamagazine.org/the-math-problem-with-pentagons-20171211/